To: "I.Vecchi" CC: Subject: Re: for the record
Date: Wed, 12 Sep 2001 22:07:51 +0100
Hi Italo,
Sorry about the prolonged silence and thanks for your messages - I've been on holiday and busy with other matters. I shall reply to your earlier comments soon (when I've retrieved them from my old computer - new technology does have its limitations!). In the meantime I'm quite happy for our, rather inconclusive, exchange to be posted on a public website - although I doubt many will find it illuminating for reasons I will explain later.
Best wishes,
Jim.
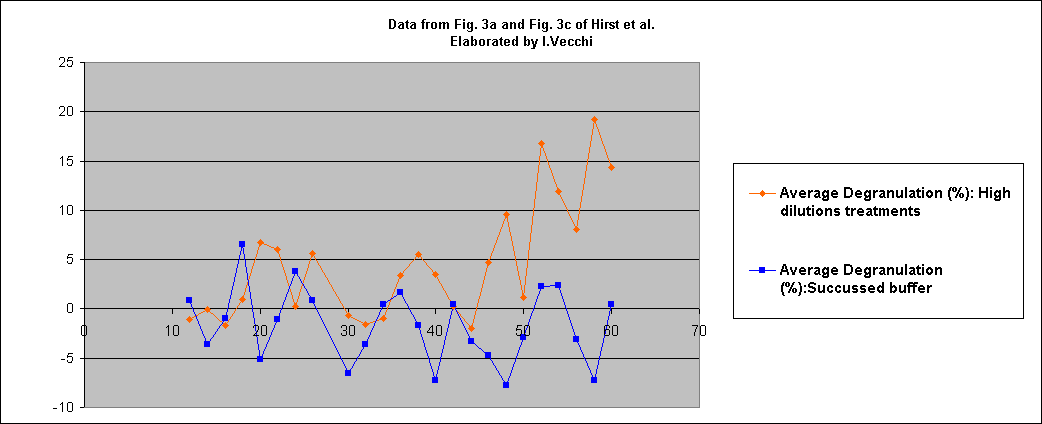
From: "Jim Burridge" <jim.burridge@btinternet.com> To: <vecchi@isthar.com> CC: <john.foreman@ucl.ac.uk> Subject: Re: for the record Date: Sun, 16 Sep 2001 23:14:06 +0100 Hi Italo, I think you are clutching at straws. I also think you and your anonymous statistical friend should study carefully my original report written 9 years ago ("A Repeat of the 'Benveniste' Experiment: Statistical Analysis", Research Report No. 100, Department of Statistical Science, University College London, England, March 1992). That report gives rather more details of the conduct of the experiment reported in Hirst et al and considerably more discussion of the statistical issues involved. The basic problem with this type of experiment is the variability of both the cell counts and the underlying biological material so any analysis is bound to have a significant statistical component. I look forward to receiving from you a reference to the detailed statistical report on which the original paper by Davenas et al is presumably based - and a copy of the raw source data would be nice too. It may well be true, as the other "JB" says, that I "couldn't understand some of it". However, he does not say what he means by "it" - does he mean his paper, his results, his statistical analysis, or your comments in this recent exchange? I cannot tell. Perhaps it doesn't matter. Incidentally, I ought to correct the emphasis of something I said in one of my earlier replies to you (e-mail dated 25 July 2001). When I wrote that I had forgotten the details of some of my discussions with the experimenters (I have since re-read my original report and refreshed my memory). I refer to the "batch" effect that I said was confounded with the high dilution treatments (incidentally, I am at a total loss as to why you think that is irrelevant - especially since some of your later comments make exactly the point that I was making!). The batch effect is a possible explanation for the results we reported - but the cause (if it is not the treatments!) is obscure for the reasons stated in my original report. Another possibility is that the results are a result of using an inappropriate statistical test - again this possibility is discussed in my original report. Perhaps you could read that report and suggest a more appropriate statistical test? There is, of course, the possibility that the results are just a chance result ....... Jim Burridge. From: I.Vecchi To: jim.burridge@btinternet.com Sent: Monday, September 17, 2001 2:02 PM Subject: Re: for the record Hi Jim, Thank you for your reply and for your suggestions. < I look forward to receiving from you a reference to the detailed statistical report on which the original paper by Davenas et al is presumably based - and a copy of the raw source data would be nice too. > The data-analysis in Davenas et al. is indeed , to put it mildly, confusing. However the effect that they claim to have detected is remarkably similar to the moderate increase in average degranulation and the strong increase in variation that you respectively dismiss as a "chance result" and attribute to an "unknown variation source". <I am at a total loss as to why you think that is irrelevant - especially since some of your later comments make exactly the point that I was making!). […] The batch effect is a possible explanation for the results we reported - but the cause (if it is not the treatments!) is obscure for the reasons stated in my original report. Another possibility is that the results are a result of using an inappropriate statistical test - again this possibility is discussed in my original report. > The origin of your results may well be a batch effect or a statistical fluke. It may also be a miracle by the Virgin Mary. You "think, but cannot prove" that it is a batch effect. What you, I, Dr. Benveniste, or the pope "think, but cannot prove" is scientifically irrelevant. Conjectures that cannot be verified/refuted are scientifically irrelevant.As I wrote previously, you are testing a null-hypothesis. If your data are incompatible with it, you should either reject the null-hypothesis or, if you realise that your method may be flawed, modify your experiment, as my statistician friend suggests, so as to eliminate the ambiguity between batch effect, statistical fluke or whatever and violation of the null-hypothesis. If such ambiguity cannot be removed, i.e. if your "batch effect" pops up whenever the null-hypothesis is being tested, then you are just calling Benveniste's "high dilution effect" by another name. Essentially you are saying " I find results which are incompatible with the null-hypothesis , so they are either chance results (as in Table 1) or (Table 2) there must be a variation source compatible with the null-hypothesis somewhere". This is not a scientific way to analyse data . Although I am fully aware that criticising an experiment is far easier than conducting one. I believe that the above points are worth making, <Perhaps you could read that report and suggest a more appropriate statistical test? There is, of course, the possibility that the results are just a chance result > I am extremely interested in reading your report. Perhaps you could send me a copy? My address is: Italo Vecchi XXXXXXXXXXXXXXXXXX XXXXXXXXXXXXXX Italy If you cannot send me a copy, I hope I can get one through the library system. In any case I will write you again after examining it. Regards, Italo Vecchi From: "Jim Burridge" <jim.burridge@btinternet.com> To: "I.Vecchi" <vecchi@isthar.com> CC: "Prof John Foreman" <john.foreman@ucl.ac.uk> Subject: Re: for the record Date: Mon, 17 Sep 2001 22:44:22 +0100 Thanks for your address - I'll send you a photocopy of my report as soon as I can. I'll comment on your thoughts about science and statistics separately, but I fear we will end up having to agree to differ. Jim. To: jim.burridge@btinternet.com Cc: john.foreman@ucl.ac.uk Sent: Wednesday, September 26, 2001 9:49 PM Subject: report Hi Jim, Thank you again for sending me your report, which I received on Monday. Here are my comments after a first reading. First of all, I noticed that your report contains no raw data. It is unfortunate that, arguably with one exception, none of the papers published on this issue contains, to my knowledge, clearly presented and complete raw experimental data. The possible exception is Benveniste et al. ("L’agitation de solutions hautement diluees … " C.R. Acad. Sci. Paris , t. 312, Serie II, 461-466, 1991) , which was co-authored, perhaps crucially, by Spira. The lack of clearly presented and complete data is, obviously and by any standard, a very serious flaw for any scientific work. I may add that the fact that you, in a previous message, apparently invoke the example of Davenas et al. as a reason for the unavailability of your raw data is somewhat perplexing. In a previous message you kindly solicited suggestions on a more appropriate statistical test. I will forward to you any further input from my statistician friend , but in the meantime you may well consider her previously posted comments. As I have stated previously, I do not understand on what basis you attribute the results in Table 2 to a batch effect, unless "batch effect" is just a more palatable name than "high dilution effect". Let me add a further remark on Benveniste et al. (see above), whose procedure has at least the merit of simplicity and may provide a good starting point for further discussion. In Benveniste et al. "experiments that could not yield valid data" are discarded "according to predefined exclusion criteria", something that you do not do in Hirst el al. , and that may explain why the effect that you dismiss as a "chance result" is possibly weaker than what they observe. On the other hand the "predefined exclusion criteria" are , in my opinion, a very delicate point, where bias may slip in one way or another. Actually one may argue that the published data in Benveniste et al. should not be considered complete, since they do not include the experiments that were discarded according to "predefined exclusion criteria". In your report, after some cautionary words about missing features of your experimental design (i.e. the fact that the <linking [of the tubes] was not recorded>), which <might have helped us interpret some of the findings described there>, i.e. the results conflicting with the null-hypothesis, you write that <However the main aim of the experiment is to show that the results do in fact behave as expected!>. Well, this may be your aim, and your honesty in stating it clearly is commendable, but I doubt that it should be the purpose of a scientific experiment. I suggest that a more appropriate aim would be "to verify whether the result are compatible with the null-hypothesis being tested". The purpose of an unbiased experiment is to obtain and weigh evidence, not to fulfil the experimenter's expectations. It is regrettable that your remarkably honest statement of purpose did not make it to the published version (i.e. Hirst et al.), since showing "that the results do in fact behave as expected!" appears indeed to be your goal. As my statistician friend points out, if you split up and multiply the tests so as to reduce the degrees of freedom, there will be little chance to detect any significant effect. The likelihood of a type II error, i.e. of wrongly accepting a false null-hypothesis, is not even considered in your discussion. Finally you literally turn the purpose of any statistical analysis on its head by dismissing the statistically significant (and Bonferroni adjusted!) effect that shows up in Table 1 as a "chance result". In your discussion of the results Table 2, where the number of degrees of freedom is higher and the anomalies are simply too strong (and perhaps unexpected) to be ignored, there are some interesting statements, which I could not find in the main paper: <one interpretation [of the results] is that there are, after all, differences between the treatments>, i.e. that, after all, Benveniste’s main claim is correct, and that <further work needs to be done>. If further work has been done, I would be grateful for any indication concerning it, since, after reading your report, I still believe that Hirst et al. provides significant, while by no means conclusive, evidence that high dilutions effects are real. Your report, while not differing substantially from the published version , is a precious contribution to this discussion, and, in my opinion, a more honest piece of work than Hirst et al.. I will make it available to some interested people, e.g. my statistician friend. I will also consider sending it to anyone requesting it by mail at my address above. Regards, tito Hi Jim, I thought you might find this interesting. The following diagram, which is based on the data in the two tables available at htttp://www.weirdtech.com/sci/hirstdata.html may help visualize the core issue. The data in the two tables correspond to the y-coordinate (in tenths of millimeter) of the points in Fig 3a and Fig 3c in Hirst et al., where, as stated therein, each point is the mean of the triplicate determinations in a single experiment. The points were measured by me using a rule. The accuracy of my measurements (or lack thereof) can be verified by anyone with some goodwill and a rule. My measurement endeavour was triggered by the adamant refusal of Hirst et al. to make their raw data available to public scrutiny. A millimeter corresponds approximately to 0,41 percentage points, hence the formula: mean average degranulation = sum(height of single points)/(5*4,1) and sum(height of single points)/(3*4,1) for high dilutions and succussed buffer respectively. The reader can decide whether the data, as plotted herewith, provide supporting evidence for Jacques Benveniste`s claims on "waves caused by extreme dilution". The most unexpected feature of the plot is the apparent periodicity in basophils degranulation in the succussed buffer. Such an effect may well be an optical fluke or whatever. If the effect is real however, then periodicity may be an intrinsic property of basophil degranulation, while highly diluted treatments increase variation and average degranulation. The time structure of measurements (i.e. basophil counts), , which has never been considered in the experimental setting, may be crucial: basophils may always subsist as an oscillating superposition between degranulating and non-degranulating state, along the lines proposed in my high-dilutions quantum model (i.e. me, see http://www.weirdtech.com/sci/feynman.html ). Highly diluted treatments may just boost the amplitude of the degranulating state as revealed by increased variation and mean. Regards, tito From : "Jim Burridge" <jim.burridge@btinternet.com> To : <vecchi@isthar.com> CC : "Prof John Foreman" <john.foreman@ucl.ac.uk> Subject : Re: "new" data on Benveniste's stuff Date : Mon, 26 Nov 2001 22:11:43 -0000 Hello again Tito! I suggest you ask yourself what would you expect random data to look like. If you can't answer that question I suggest you take the next logical step and use your favourite stats package (or even Microsoft Excel will do) to produce 24 random standard normal numbers and plot them against the order in which they were produced (not in order of magnitude of course!). Ask yourself whether the plot looks periodic or not. Of course you might, just by chance, produce an increasing sequence...........! Try it a few, or even several times, and you might appreciate the difficulty. Best wishes, Jim
From: "I.Vecchi"
To: "jim.burridge@btinternet.com"
Subject: "new" data on Benveniste's stuff